Find Log

Published: 19 Aug 2023

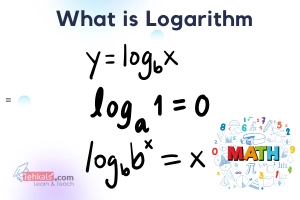
Logarithms are powerful mathematical tools with applications across various scientific, engineering, and mathematics. Whether solving complex equations, analyzing exponential form, or working with complicated calculations, understanding how to find log is crucial. To find log is difficult to find and solve, sol these rules are explained and simplified in the article “How to find log”.

How to Find Log

It is difficult to find log of base-10 for learners and students.

Common Logarithm

The common logarithm was invented by a British Mathematician, Prof. Henry Briggs (1560-1631).

Definition of Common Logarithm

Logarithms having base $ 10 $ are called common logarithms or Briggs logarithms.
Note:
The base of the logarithm is not written because it is considered to be $ 10 $.

Parts of a common logarithm

The logarithm of the number consists of two parts.

Characteristics

The digit before the decimal point or Integral part is called the characteristics.

Mantissa

The decimal fraction part is the mantissa.

Example:

$ 1.5377 $
In this example
$ Characteristics=1 $
$ Mantissa=.5377 $

Log Table

The log table is organized into columns and provides a convenient way to find the logarithm of a given number.

Parts of Log Table

A logarithm table is divided into three parts.

Main Column

The first part of the table, the main column, contains numbers from $ 10 \ to \ 99 .$

Differences columns

The second part of the table, called differences columns, consists of $ 10 $ columns headed by $ 0, 1, 2, …. 9 $

Mean Differences

The third part consists of small columns known as mean differences headed by $ 1, 2, 3, … 9 $.

How to use a Log Table

  • First, take the two digits of the number whose logarithm is required and see it in the row of the main column of the log table.
  • Proceed horizontally along the selected row till the column headed by the third digit. The number under these columns is taken to find mantissa.
  • Now see the fourth digit in the mean differences columns of the same row and add to the mantissa found in the second column.

How To Find Mantissa

Here are the steps to find mantissa with example and explanation.

Example to Find Mantissa

$ 432.5 $
Solution:

  • First, ignore the decimal point.
  • Take the first two digits, e.g. $ 43 $ and proceed along this row until we come to the column headed by the third digit $ 2 $ of the number, which is $ 6355 $.
  • Now take the fourth digit, i.e. $ 5 $ and proceed along this row in the mean difference column, which is $ 5 $.
  • Now add $ 6355+5=6360 $

How to find the common logarithm

  • Round off the given number to four significant figures.
  • Find the characteristics of the logarithm.
  • Find the Mantissa from the log table.
  • Combine Characteristics and Mantissa.
Find logarithms of $ 2476 $

Solution:
$ 2476 $
Let $ x=2476 $
Taking log on B.S
$ log \ ⁡ x=log \ ⁡2476 $
In Scientific form:
$ 2.476 \times 10^3 $
$ Thus \ Characteristics =3 $
To find Mantissa, using Log Table
$ Mantissa =.3938 \quad \ As 3927+11 $
Hence $ log \ ⁡2476=3.3938 $

Logarithm – MCQs

1. Logarithms having base 10 are called________ Logarithms
O Natural
O Common
O Briggs
O Both b & c
Show Answer

Common
Explanation:


2. Common logarithm is also called __________ logarithm
O Natural
O Briggs
O Both a & b
O None of these
Show Answer

Briggs
Explanation:


3. The digit before the decimal point or integral part is called _____________
O Characteristics
O Mantissa
O Both a & b
O None of these
Show Answer

Characteristics
Explanation:
In $ 1.5377 $ Characteristics is 1.


4. The decimal fraction part is called ________
O Characteristics
O Mantissa
O Both a & b
O None of these
Show Answer

Mantissa
Explanation:
In $ 1.5377 Mantissa \ is \ .5377 $.


5. In $ 1.5377 $, characteristics is
O 1
O $ .5377 $
O $ 1.5377 $
O None of these
Show Answer

1
Explanation:
The digit before the decimal point or Integral part is called characteristics.


6. In $ 1.5377 $, Mantissa is
O 1
O $ .5377 $
O $ 1.5377 $
O None of these
Show Answer

$ .5377 $
Explanation:
The decimal fraction part is Mantissa.


7. The mean difference digits are added to ______________
O Characteristics
O Mantissa
O Both a & b
O None of these
Show Answer

Mantissa
Explanation:
The mean difference is the third part to find the mantissa and it is added to mantissa.


8 The mantissa of $ 763.5 $ is
O $ .8825 $
O $ .8828 $
O 2
O 76
Show Answer

$ .8828 $
Explanation:
(i). First ignore the decimal point
(ii). Take first two digits e.g. 76 and proceed along this row until we come to column headed by third digit 3 of the number which is 8825
(iii). Now take fourth digit i.e. 5 and proceed along this row in mean difference column which is 5.
(iv). Now add $ 8825+3=8828 $
Thus Mantissa of $ 763.5 \ is \ .8828 $


9. The characteristics of 982.5 is
O 0
O 2
O 3
O 4
Show Answer

2
Explanation:
First convert 982.5 to Scientific form:
$ 9.825 \times 10^2 $
Thus Characteristics is 2


10. The characteristics of 7824 is
O 0
O 1
O 2
O 3
Show Answer

3
Explanation:
First convert 7824 to Scientific form:
$ 7.824 \times 10^3 $
Thus Characteristics is 3


11. The characteristics of 56.3 is
O 0
O 1
O 2
O 3
Show Answer

1
Explanation:
First convert 56.3 to Scientific form:
$ 5.63 \times 10^1 $
Thus Characteristics is 1


12. The characteristics of 7.43 is
O 0
O 1
O 2
O 3
Show Answer

0
Explanation:
First convert 7.43 to Scientific form:
$ 7.43 \times 10^0 $
Thus Characteristics is 0


13. The characteristics of 0.71 is
O 1
O $ -1 $
O 2
O $ -2 $
Show Answer

$ -1 $
Explanation:
First convert 0.71 to Scientific form:
$ 7.1 \times 10^{-1} $
Thus Characteristics is $ -1 $


14. The characteristics of 37300 is
O 0
O 2
O 3
O 4
Show Answer

4
Explanation:
First convert 37300 to Scientific form:
$ 3.73 \times 10^4 $
Thus Characteristics is 4


15. The characteristics of $ 0.00159 $ is
O 1
O $ -1 $
O $ -3 $
O $ -2 $
Show Answer


Explanation:
First convert $ 0.00159 $ to Scientific form:
$ 0.00159 \times 10^{-3} $
Thus Characteristics is $ -3 $


16. The mantissa of 2476 is
O $ .3927 $
O $ .3938 $
O 3
O None of these
Show Answer


Explanation:
(i). First ignore the decimal point
(ii). Take first two digits e.g. 24 and proceed along this row until we come to column headed by third digit 7 of the number which is 3927
(iii). Now take fourth digit i.e. 6 and proceed along this row in mean difference column which is 11.
(iv). Now add $ 3927+11=3938 $
Thus Mantissa of $ 2476 \ is \ .3938 $


17. The log of 2.4 is
O 24
O 0.3802
O 2.3802
O None of these
Show Answer

0.3802
Explanation:
See Ex # 3.3
Q No. 3
Part No. (ii)


18. The log of 482.7 is
O .6836
O 2.6836
O 2.6830
O None of these
Show Answer

2.6836
Explanation:
See Ex # 3.3
Q No. 3
Part No. (iv)


19. The log of 0.783 is
O $ .8938 $
O $ \overline{1} .8938 $
O 1.8938
O None of these
Show Answer

$ \overline{1} .8938 $
Explanation:
See Ex # 3.3
Q No. 3
Part No. (v)


20. The log of 0.09566 is
O $ \overline{2} .9805 $
O $ \overline{2} .9808 $
O 2.9808
O None of these
Show Answer

$ \overline{2} .9808 $
Explanation:
See Ex # 3.3
Q No. 3
Part No. (vi)


21. The log of 700 is
O .8451
O 1.8451
O 2.8451
O None of these
Show Answer

2.8451
Explanation:
See Ex # 3.3
Q No. 3
Part No. (viii)

jawad khalil Avatar
jawad khalil
Please Write Your Comments
Comments (0)
Leave your comment.
Write a comment
INSTRUCTIONS:
  • Be Respectful
  • Stay Relevant
  • Stay Positive
  • True Feedback
  • Encourage Discussion
  • Avoid Spamming
  • No Fake News
  • Don't Copy-Paste
  • No Personal Attacks
`