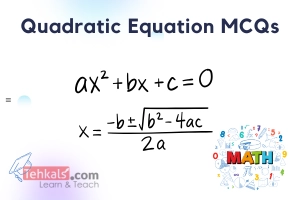
Chapter 2 Theory of Quadratic Equation
Published: 9 Apr 2023
In Chapter 2, maths class 10, students will learn about the theory of quadratic equations (Discriminant of quadratic equations & Cube Roots of Unity). This chapter also includes multiple-choice questions (MCQs of Chapter 2 Mathematics) to learn and test their understanding of the material to secure good marks.
Chapter 2 Maths Class 10 MCQs
Exercise # 2.1 MCQs
Discriminant of a Quadratic Equation
In this portion of Chapter 2 Maths Class 10 includes the MCQs of Discriminant of a Quadratic Equation.
1. In quadratic formula, the expression $ b^{2}-4 a c $ is called ________ of quadratic equation.(a) Factorization
(b) Functions
(c) Discriminant
(d) Irrational
Show Answer
Discriminant
2. The value of the discriminant is used to determine the number of solutions of a ________ equation.
(a) Linear
(b) Quadratic
(c) Simultaneous
(d) None of these
Show Answer
Quadratic
3. If $ b^{2}-4 a c $ ________ then the roots are real, equal and rational.
(a) $ =0 $
(b) <0
(c) >0
(d) None of these
Show Answer
$ =0 $
4. If $ b^{2}-4 a c $ ________ then the roots are unequal and imaginary.
(a) $ =0 $
(b) <0
(c) >0
(d) None of these
Show Answer
<0
5. If $ b^{2}-4 a c $ ________ and root is a perfect square, then roots are real, unequal and rational.
(a) $ =0 $
(b) <0
(c) >0
(d) None of these
Show Answer
Answer: $ >0 $
6. If $ b^{2}-4 a c $ ________ and root is not a perfect square, then roots are real, unequal and irrational.
(a) $ =0 $
(b) <0
(c) >0
(d) None of these
Show Answer
Answer: $ >0 $
7. If $ b^{2}-4 a c>0 $ and roots are perfect square then roots are ________
(a) Rational
(b) Irrational
(c) Equal
(d) Imaginary
Show Answer
Rational
8. If $ b^{2}-4 a c>0 $ and roots are not perfect square then roots are ________
(a) Rational
(b) Irrational
(c) Equal
(d) Imaginary
Show Answer
Irrational
9. The discriminant of $ x^{2}+9 x+2=0 $
(a) $ -73 $
(b) 73
(c) 0
(d) $ 9 x+2 $
Show Answer
Answer: 73
Explanation:
$ x^{2}+9 x+2=0 $
Compare it with $ a x^{2}+b x+c=0 $
$ Here a=1, b=9, c=2 $
As we have
$ Discriminant =b^{2}-4 a c $
$ Discriminant =(9)^{2}-4(1)(2) $
$ Discriminant =81-8 $
$ Discriminant =73 $
Nature of Roots
10. What is the nature of the roots of $ x^{2}-8 x+16=0 $(a) Real, equal and rational
(b) Real, unequal and irrational
(c) Real, unequal and rational
(d) Unequal and imaginary
Show Answer
Answer: Real, equal and rational
Explanation:
$ x^{2}-8 x+16=0 $
Compare it with $ a x^{2}+b x+c=0 $
$ Here a=1, b=-8, c=16 $
As we have
$ Discriminant =b^{2}-4 a c $
$ Discriminant =(-8)^{2}-4(1)(16) $
$ Discriminant =64-64 $
$ Discriminant =0 $
Thus the roots are real, equal and rational
11. What is the nature of the roots of $ x^{2}+9 x+2=0 $
(a) Real, equal and rational
(b) Real, unequal and irrational
(c) Real, unequal and rational
(d) Unequal and imaginary
Show Answer
Answer: Real, unequal and irrational
Explanation:
$ x^{2}+9 x+2=0 $
Compare it with a $ x^{2}+b x+c=0 $
$ Here a=1, b=9, c=2 $
As we have
$ Discriminant =b^{2}-4 a c $
$ Discriminant =(9)^{2}-4(1)(2) $
$ Discriminant =81-8 $
$ Discriminant =73>0 $
Thus the roots are real, unequal and irrational
12. What is the nature of the roots of $ 6 x^{2}-x-15=0 $
(a) Real, equal and rational
(b) Real, unequal and irrational
(c) Real, unequal and rational
(d) Unequal and imaginary
Show Answer
Answer: Real, unequal and rational
Explanation:
$ 6 x^{2}-x-15=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=6, b=-1, c=-15 $
As we have
$ Discriminant =b^{2}-4 a c $
$ Discriminant =(-1)^{2}-4(6)(-15) $
$ Discriminant =1+360 $
$ Discriminant =361 $
$ Discriminant =19^{2}>0 $
Thus the roots are real, unequal and rational
13. What is the nature of the roots of $ 4 x^{2}+x+1=0 $
(a) Real, equal and rational
(b) Real, unequal and irrational
(c) Real, unequal and rational
(d) Unequal and imaginary
Show Answer
Answer: Unequal and imaginary
Explanation:
$ 4 x^{2}+x+1=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=4, b=1, c=1 $
As we have
$ Discriminant =b^{2}-4 a c $
$ Discriminant =(1)^{2}-4(4)(1) $
$ Discriminant =1-16 $
$ Discriminant =-15 $<0
Thus the roots are unequal and imaginary
14. Without solving, determine the nature of the roots of the $ 3 x^{2}-4 x+6=0 $
(a) Real, equal and rational
(b) Real, unequal and irrational
(c) Real, unequal and rational
(d) Unequal and imaginary
Show Answer
Answer: Unequal and imaginary
Explanation:
$ 3 x^{2}-4 x+6=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=3, b=-4, c=6 $
As we have
$ Discriminant =b^{2}-4 a c $
$ Discriminant =(-4)^{2}-4(3)(6) $
$ Discriminant =16-72 $
$ Discriminant =-56 $<0
Thus the roots are unequal and imaginary
15. Determine the value of $ {k} $ for which the given quadratic equation have real roots.
$ k x^{2}+4 x+1=0 $
(a) k>4
(b) k<4
(c) $ k \geq 4 $
(d) $ k \leq 4 $
Show Answer
Answer:
Explanation:
$ k x^{2}+4 x+1=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=k, b=4, c=1 $
If roots are Real
$ Discriminant =b^{2}-4 a c \geq 0 $
$ b^{2}-4 a c \geq 0 $
$ (4)^{2}-4(k)(1) \geq 0 $
$ 16-4 k \geq 0 $
$ 16 \geq 4 k $
$ \frac{16}{4} \geq k $
$ 4 \geq k $
$ k \leq 4 $
16. For what value of $ {k} $ the roots of the following equation are imaginary $ 2 x^{2}+3 x+k=0 $
(a) k>4
(b) k<4
(c) $ k \leq \frac{9}{8} $
(d) $ k>\frac{9}{8} $
Show Answer
Answer: k>$ \frac{9}{8} $
Explanation:
$ 2 x^{2}+3 x+k=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=2, b=3, c=k $
If roots are Imaginary
$ Discriminant =b^{2}-4 a c $<0
$ b^{2}-4 a c $<0
$ (3)^{2}-4(2)(k) $<0
$ 9-8 k $<0
9<8k
$ \frac{9}{8} $
17. Quadratic equation $ ax^2+bx+c=0 $ has equal roots if $ b^2-4ac= $
(a) $ =0 $
(b) <0
(c) >0
(d) None of these
Show Answer
$ =0 $
Exercise # 2.2 MCQs
In this exercise of Chapter 2 Maths Class 10 includes the MCQs of Cube Root of Unity.
Cube Root of Unity
Exercise # 2.3 MCQs
Sum and Product of Roots
In this portion of Chapter 2 Maths Class 10 includes the MCQs Sum of Roots and Product of Roots.
1. Sum of roots $ = $(a) $ \frac{-b}{a} $
(b) $ \frac{c}{a} $
(c) $ \omega $
(d) $ \omega^{2} $
Show Answer
Answer: $ \frac{-b}{a} $
2. Product of roots $= $
(a) $ \frac{-b}{a} $
(b) $ \frac{c}{a} $
(c) $ \omega $
(d) $ \omega^{2} $
Show Answer
Answer: $ \frac{c}{a} $
3. Sum of the roots of $ 2 x^{2}-3 x-4=0 $
(a) $ \frac{3}{2} $
(b) $ -2 $
(c) $ \omega $
(d) $ \omega^{2} $
Show Answer
Answer: $ \frac{3}{2} $
Explanation:
$ 2 x^{2}-3 x-4=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=2, b=-3, c=-4 $
Let $ \alpha \ and \ \beta$ be the roots of equation
Then sum of roots:
$ \alpha+\beta=\frac{-b}{a} $
$ \alpha+\beta=\frac{-(-3)}{2} $
$ \alpha+\beta=\frac{3}{2} $
4. Products of the roots of $ 2 x^{2}-3 x-4=0 $
(a) $ \frac{3}{2} $
(b) $ -2 $
(c) $ \omega $
(d) $ \omega^{2} $
Show Answer
Answer: $ -2 $
Explanation:
$ 2 x^{2}-3 x-4=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=2, b=-3, c=-4 $
Let $ \alpha \ and \ \beta $ be the roots of equation
Product of roots:
$ \alpha \cdot \beta=\frac{c}{a} $
$ \alpha \cdot \beta=\frac{-4}{2} $
$ \alpha \cdot \beta=-2 $
Exercise # 2.4 MCQs
In this Exercise of Chapter 2 Maths Class 10 includes the MCQs of Symmetric Functions of Roots of a Quadratic Equation.
5. Let $ \alpha, \beta $ be the roots of a quadratic equation, then the expressions of the form of $ \alpha+\beta, \alpha \beta, \alpha^{2}+\beta^{2} $ are called the _________ of the roots of the quadratic equation.(a) Solutions
(b) Roots
(c) Functions
(d) None of these
Show Answer
Answer: Functions
6. By _________ function of the roots of an equation, we mean that the function remains unchanged in values when the roots are interchanged.
(a) Solution
(b) Root
(c) Symmetric
(d) None of these
Show Answer
Answer: Symmetric
7. The functions $ \alpha+\beta, \alpha^{2}+\beta^{2}, \alpha^{3}+\beta^{3} $ are _________ function of $ \alpha \ and \ \beta $
(a) Solution
(b) Root
(c) Symmetric
(d) None of these
Show Answer
Answer: Symmetric
Explanation:
By Symmetric function of the roots of an equation, we mean that the function remains unchanged in values when the roots are interchanged.
8. $ {\alpha}^{2}+{\beta}^{2}= $
(a) $ (\alpha+\beta)^{2} $
(b) $ (\alpha+\beta)^{2}-2 \alpha \beta $
(c) $ (\alpha-\beta)^{2}-2 \alpha \beta $
(d) None of these
Show Answer
Answer: $ (\alpha+\beta)^{2}-2 \alpha \beta $
Explanation:
As
$ \alpha^{2}+\beta^{2}+2 \alpha \beta=(\alpha+\beta)^{2} $
$ \alpha^{2}+\beta^{2}=(\alpha+\beta)^{2}-2 \alpha \beta $
Then
$ \alpha^{2}+\beta^{2}=(\alpha+\beta)^{2}-2 \alpha \beta $
9. $ \alpha^{3}+{\beta}^{3}= $
(a) $ (\alpha+\beta)^{3} $
(b) $ (\alpha+\beta)^{3}-3 \alpha \beta $
(c) $ -3 \alpha \beta(\alpha+\beta) $
(d) $ (\alpha+\beta)^{3}-3 \alpha \beta(\alpha+\beta) $
Show Answer
Answer: $ (\alpha+\beta)^{3}-3 \alpha \beta(\alpha+\beta) $
Explanation:
As
$ \alpha^{3}+\beta^{3}+3 \alpha\beta(\alpha+\beta)=(\alpha+\beta)^{3} $
Then
$ \alpha^{3}+\beta^{3}=(\alpha+\beta)^{3}-3 \alpha \beta(\alpha+\beta) $
10. $ \frac{1}{\alpha}+\frac{1}{\beta}= $
(a) $ \frac{\alpha+\beta}{\alpha \beta} $
(b) $ \beta+\alpha $
(c) $ \beta \alpha $
(d) $ \alpha+\beta $
Show Answer
Answer: $ \frac{\alpha+\beta}{\alpha \beta} $
Explanation:
$ \frac{1}{\alpha}+\frac{1}{\beta}=\frac{\beta+\alpha}{\alpha \beta} $
$ \frac{1}{\alpha}+\frac{1}{\beta}=\frac{\alpha+\beta}{\alpha \beta} $
11. If S and P be the sum and product of roots of a quadratic equation respectively, then the quadratic equation is
(a) $ x^2+Sx+P=0 $
(b) $ x^2-Sx+P=0 $
(c) $ x^2-Sx-P=0 $
(d) $ x^2+Sx-P=0 $
Show Answer
Answer: $ x^2-Sx+P=0 $
Explanation:
As $ a x^2+b x+c=0 $
Divide all terms by $a$
$ \frac{a x^2}{a}+\frac{b x}{a}+\frac{c}{a}=\frac{0}{a} $
$ x^2+\frac{b x}{a}+\frac{c}{a}=0 $
Now we can write it as
$ x^2-\left(-\frac{b}{a}\right) x+\frac{c}{a}=0 $
As $ -\frac{b}{a}=\mathrm{S} \ and \ \frac{c}{a}=\mathrm{P} $
Then
$ x^2-\mathrm{S} x+\mathrm{P}=0 $
12. Form a quadratic equation whose roots are $ 1+\sqrt{5}, 1-\sqrt{5} $
(a) $ x^{2}-\sqrt{5} x+1=0 $
(b) $ x^{2}-S x+P=0 $
(c) $ x^{2}-2 x-4=0 $
(d) None of these
Show Answer
Answer: $ x^{2}-2 x-4=0 $
Explanation:
As $ 1+\sqrt{5} and 1-\sqrt{5} $ are the roots of required equation
Then sum of roots:
$ S=1+\sqrt{5}+1-\sqrt{5} $
$ S=1+1+\sqrt{5}-\sqrt{5} $
$ S=2 $
And product of roots:
$ P=(1+\sqrt{5})(1-\sqrt{5}) $
$ P=(1)^2-(\sqrt{5})^2 $
$ P=1-5 $
$ P=-4 $
As required equation is:
$ x^2-S x+P=0 $
Now
$ x^2-2 x+(-4)=0 $
$ x^2-2 x-4=0 $
13. $ (\alpha-\beta)^{2}= $
(a) $ (\alpha+\beta)^{2} $
(b) $ (\alpha+\beta)^{2}-4 \alpha \beta $
(c) $ 2 \alpha \beta $
(d) $ 4 \alpha \beta $
Show Answer
Answer: $ (\alpha+\beta)^{2}-4 \alpha \beta $
Explanation:
$ (\alpha-\beta)^{2} $
$ =(\alpha+\beta)^{2}-4 \alpha \beta $
14. ___________ division is the process of finding the quotient and remainder with less writing and fewer calculations.
(a) Synthetic
(b) Long
(c) No
(d) None of these
Show Answer
Answer: Synthetic
15. ___________ division is the shortcut of long division method and allows one to calculate without writing variables.
(a) Synthetic
(b) Long
(c) No
(d) None of these
Show Answer
Answer: Synthetic
16.___________ division can be used only when the divisor is a linear factor.
(a) Synthetic
(b) Long
(c) No
(d) None of these
Show Answer
Answer: Synthetic
17. More than one equation which are satisfied by the same values of the variables involved are called ___________ equations.
(a) Quadratic
(b) Linear
(c) Simultaneous
(d) None of these
Show Answer
Answer: Simultaneous
18. A system of Linear equation consists of two or more ___________ equations in the same variables.
(a) Quadratic
(b) Linear
(c) Function
(d) None of these
Show Answer
Answer: Linear
19. The sum of roots of an equation $ x^2-5kx++6k^2=0 $ is
(a) $ 5k $
(b) $ -5k $
(c) $ 6k^2 $
(d) None of these
Show Answer
Answer: $ 5k $
Explanation:
$ x^2-5kx++6k^2=0 $
Compare it with $ a x^{2}+b x+c=0 $
Here $ a=1, b=-5k, c=6k^2 $
Let $ \alpha \ and \ \beta $ be the roots of equation
Then sum of roots:
$ \alpha+\beta=\frac{-b}{a} $
$ \alpha+\beta=\frac{-(-5k)}{1} $
$ \alpha+\beta=5k $
Review Chapter 2 Mahts Class 10
(i). If the sum of roots of$ (a+1)x^2+(2a+3)x+(3a+4)=0 $
is $ -1 $, then roots is
O 0
O 1
O 2
O 3
Show Answer
2
Explanation:
$ (a+1)x^2+(2a+3)x+(3a+4)=0 $
$ a=a+1, b=2a+2, c=3a+4 $
As
Sum of roots$ =s=-1 $
Now
$ S=\frac{-b}{a} $
$ -1=\frac{-(2a+3)}{a+1} $
$ -1(a+1)=-2a-3 $
$ -a-1=-2a-3 $
$ -a+2a=-3+1 $
$ a=-2 $
Now
$ P=\frac{c}{a} $
$ P=\frac{3a+4}{a+1} $
Put $ a=-2 $
$ P=\frac{3(-2)+4}{-2+1} $
$ P=\frac{-6+4}{-1} $
$ P=\frac{-2}{-1} $
$ P=2 $
(ii). The sum of the roots of a quadratic equation is 2 and the sum of the cubes of the roots is 98. The equation is
O $ x^2-2x-15=0 $
O $ x^2-2x+15=0 $
O $ x^2-4x+15=0 $
O None of these
Show Answer
$ x^2-2x-15=0 $
Explanation:
Let $ \alpha, \beta $ be the roots of quatratic eaquation
As we know that
$ S=\alpha+ \beta $
$ P=\alpha \beta $
Now we have
Sum of roots
$ S=\alpha+ \beta=2 $
and sum of cumbes of the roots $ \alpha^3+ \beta^3 =98$
Now
$ (\alpha+ \beta)^3 -3(\alpha \beta)(\alpha+ \beta)=98$
$ 8 -6(\alpha \beta)=98$
$ -6(\alpha \beta)=98-8$
$ -6(\alpha \beta)=90$
$ \alpha \beta=\frac{90}{-6}$
$ \alpha \beta=-15$
So
$ P=\alpha \beta=-15$ For
As required equation is
$ x^2-Sx+P=0 $
So
$ x^2-2x+(-15)=0 $
$ x^2-2x-15=0 $
(iii). If a, b, c are positive real number, then both the roots of the equation $ a^2+bx+c=0 $, are always
O real and positive
O real and negative
O rational and unequal
O none of these
Show Answer
None of these
(iv). If $ a $ and $ b $ are the roots of $ 4x^2-3x+7=0 $ then the value of $ \frac{1}{a}+ \frac{1}{b}$ is
O $ -\frac{3}{4} $
O $ \frac{3}{7} $
O $ -\frac{3}{7} $
O $ \frac{4}{7} $
Show Answer
$ \frac{3}{7} $
Explanation:
$ 4x^2-3x+7=0 $
$ a=4, b=-3, c=7 $
Let a and b be the roots of equation
Then sum of roots:
$ a+b=\frac{-b}{a}=\frac{-(-3)}{4} $
$ a+b=\frac{3}{4} $
and Product of roots:
$ ab=\frac{c}{a}=\frac{7}{4} $
According to given condition
$ \frac{1}{a}+\frac{1}{b}=\frac{a+b}{ab} $
$ \frac{1}{a}+\frac{1}{b}=\frac{3/4}{7/4} $
$ \frac{1}{a}+\frac{1}{b}=\frac{3}{7} $

- Be Respectful
- Stay Relevant
- Stay Positive
- True Feedback
- Encourage Discussion
- Avoid Spamming
- No Fake News
- Don't Copy-Paste
- No Personal Attacks

- Be Respectful
- Stay Relevant
- Stay Positive
- True Feedback
- Encourage Discussion
- Avoid Spamming
- No Fake News
- Don't Copy-Paste
- No Personal Attacks